
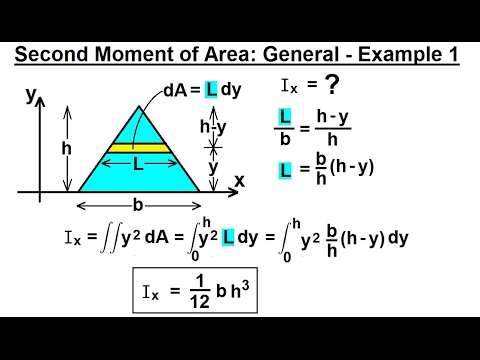
Each of these shapes will have a centroid (C) or center of mass (G) listed on the diagram. This may include areas or volumes (which we will count as positive areas or volumes) or holes (which we will count as negative areas or volumes). Next, we must break our complex shape down into several simpler shapes. It will be important to measure all locations from the same point. Start the process by labeling an origin point and axes on your shape. Finding the Centroid via the Method of Composite Parts The method of composite parts is limited in that we will need to be able to break our complex shape down entirely into shapes found in the centroid table we have available or the method will not work without also doing some moment integrals. Links to some centroid tables can be found in the sidebar to the right. This is a set of tables that lists the centroids (and usually also moments of inertia) for a number of common areas and/or volumes.
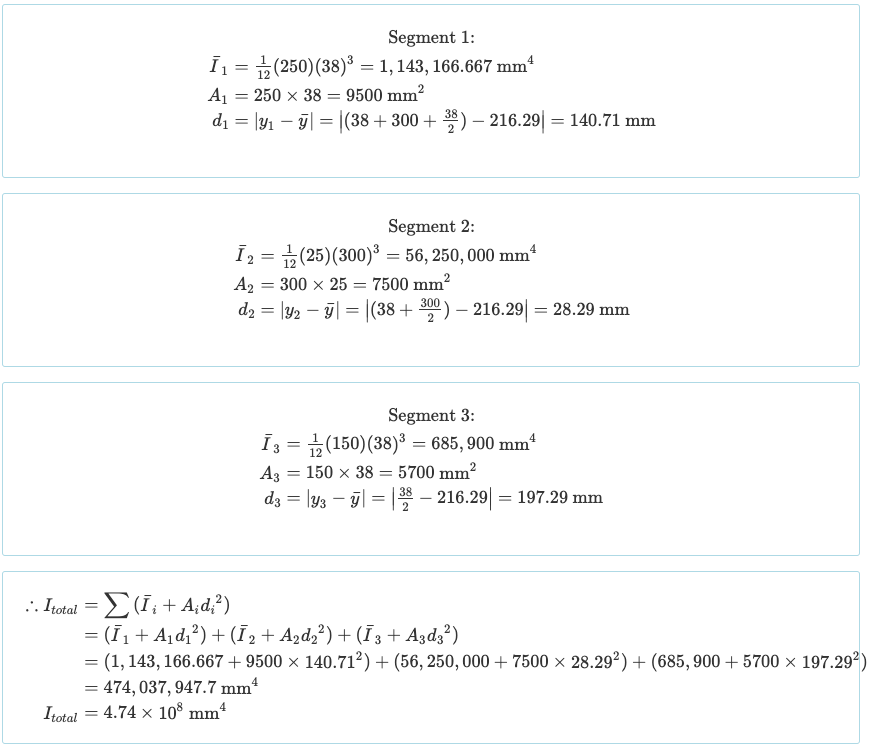
The method works by breaking the shape or volume down into a number of more basic shapes, identifying the centroids or centers of masses of each part via a table of values, and then combing the results to find the overall centroid or center of mass.Ī key aspect of the method is the use of these centroid tables. This method is often easier and faster that the integration method however, it will be limited by the table of centroids you have available.
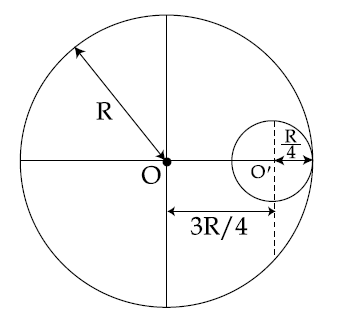

R = distance between axis and rotation mass (in.Finding the Centroid, Center of Mass and Mass Moment of Inertia via the Method of Composite PartsĪs an alternative to the use of moment integrals, we can use the Method of Composite Parts to find the centroid of an area or volume or the center of mass of a body. I = ∑ i m i R i 2 = m 1 R 1 2 + m 2 R 2 2 +. The moment of all other moments of inertia of an object are calculated from the the sum of the moments. R = distance between axis and rotation mass (ft, m) I = moment of inertia (lb m ft 2, kg m 2 ) Point mass m (mass) at a distance r from the axis of rotation. Geometrically simple objects have moments of inertia that can be expressed mathematically, but it may not be straightforward to symbolically express the moment of inertia of more complex bodies. It should not be confused with the second moment of area, which is used in bending calculations. Mass moments of inertia have units of dimension mass × length 2. The mass moment of inertia, usually denoted I, measures the extent to which an object resists rotational acceleration about an axis, and is the rotational analogue to mass. Related Resources: mechanics machines Mass Moment of Inertia Equations


 0 kommentar(er)
0 kommentar(er)
